More on Evaluating Functions

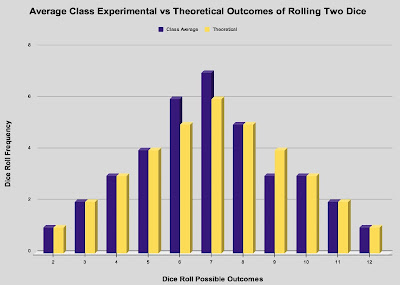
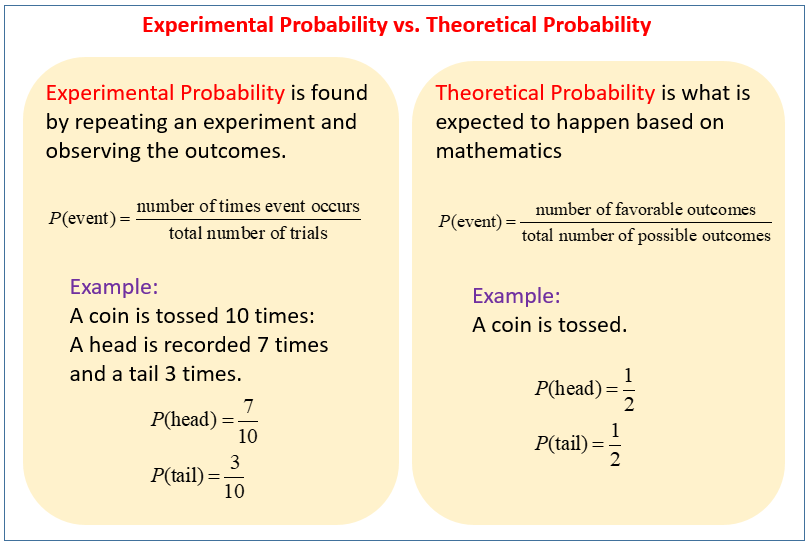
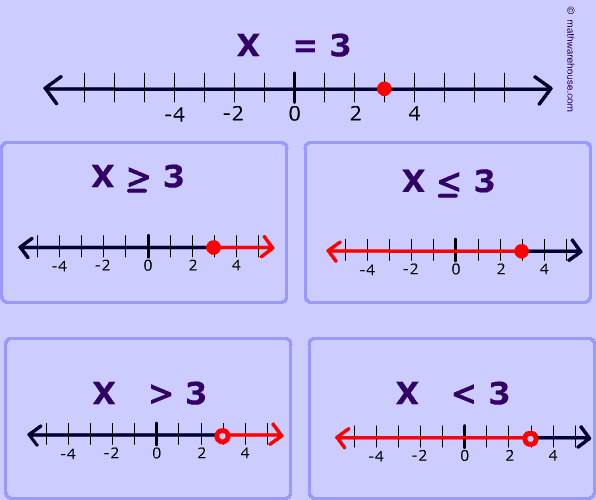
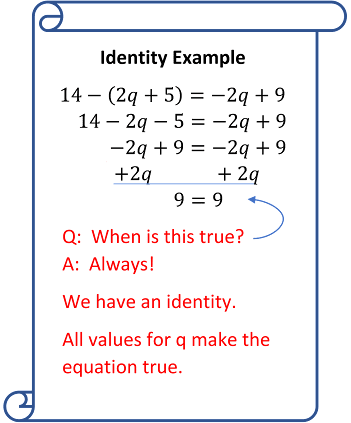
Today We Discussed:

Relations, functions, domain and range and evaluating functions are still the topic of the day.  We worked on evaluating functions using tables, and sketching the graph.  We worked more with evaluating functions for various domain (x) values that involve both numbers and variables.  We also used function notation to translate values into coordinate points and further practiced determining the domain and range of functions and relations from a set of points and from graphs. 

Graphic credit: https://www.mathbootcamps.com/function-notation-and-evaluating-functions/

Sections Covered in Textbook:

No new sections were covered in the book today. 

Resources & Tutorials:

1) Evaluating a function from a graph. (video)
2) Evaluate a function from a graph.  (online practice)

3) More on Evaluating Functions class notes

Writing a Function Rule

Today We Discussed:

We continued our discussion about functions and explored how to write a function rule (basically this is an equation) from a table of values or a graph of coordinate points.  When we move deeper into linear functions, finding the slope, and graphing, we'll take a look at how to deduce a function rule that involves more than one operation.

Sections Covered in Textbook:

5-5: Writing a Function Rule (pages 254-260)

Resources & Tutorials:

1) How do you write a rule from a table? 
2) Finding the function rule from a table (more complicated examples).

3) Writing a Function Rule class notes

Function Rules, Tables, and Graphs

Today We Discussed:

We expanded our work on functions today with an exploration of graphing.  We discussed three different ways to view a function (as an equation, as a table, and as a graph).  We played around with linear, absolute value, and quadratic functions.

One aspect of functions that is nice is that we can pick what values to use for our domain, and this is especially helpful when dealing with functions containing fractions.  We can pick numbers for the domain that multiply to give us whole numbers, to make our graphs easier to draw.  I will continue to reinforce to students to choose wisely when picking values for the domain.

We also discussed the generic shapes of the three types of functions we talked about.  Linear functions will create a line; absolute value functions will generate a "V"; quadratic equations will generate a "U" or what is called a parabola. 

Vocabulary: independent variable, dependent variable

Sections Covered in Textbook:
5-3: Function Rules, Tables, and Graphs (pages 247 - 252)

Resources & Tutorials:

1) How do you graph a linear function using a table?
2)  Graph an absolute value function from a table.
3)  Graph quadratic function from a table.
4) 6 Mini-Coordinate Planes for Graphing

5) Function Rules, Tables, and Graphs class notes

Relations and Functions Part 2

Today We Discussed:

We expanded our discussion about relations and functions today and took a look at function notation.  Most students are intimidated by this method of representing an equation in two variables.  They are mostly comfortable with an equation of the form

y = 2x + 3 

We discussed that f(x) = 2x + 3 is just a fancy way of writing the above equation, and it can be described by saying "there is some function that uses the variable x, where the function rule is 2x+3". 

We discussed evaluating functions for given domain values, to produce range values.

DIXI-ROYD was also reinforced today.

Vocabulary:   DIXI-ROYD, function rule, evaluate function, function notation

Sections Covered in Textbook:

5-2: Relations and Functions (pages 241-246)

Resources & Tutorials:

1) What is function notation?
2) How do you find f(x) if given a value for x?

3) Relations and Functions Part 2 class notes

Relations and Functions Part 1

Today We Discussed:

Today we explored what relations are, and discussed the concepts of domain and range.  We also defined functions as special relations where there is exactly one range value for each given domain value.  We modeled the different ways of representing a relation:  set of ordered pairs, table, mapping diagram, and graph, and used the vertical line test to determine if a graph is a function or not.  We also discussed why graphs that fail the vertical line test are not functions.

Many students will try to "force" a relation to be a function, or will feel like it is "bad" if a relation is not a function.  We discussed this tendency, and why it's perfectly fine to have a relation that is not a function.  Just because something does not fit a particular definition does not make it bad or good - it just is what it is!

Vocabulary:  relation, domain, range, function, vertical line test, mapping diagram, 

Sections Covered in Textbook:

5-2: Relations and Functions (pages 241-246)

Resources & Tutorials:

1) What is a relation?
2) What is domain?
3) What is the range of a relation?
4) How do you find the domain and range of a relation?
5) What is a function?
6) How do you figure out if a relation is a function?

7) Relations and Functions Part 1 class notes

Relating Events to Graphs

Today We Discussed:

We began our next unit on functions and graphs.  Today we related graphs to real events, by analyzing graphs that relate to time passing.  The shape of the graph can tell a story of what is happening as time passes.

For graphs in general, we will be talking about independent and dependent variables.  Time is considered an independent variable with other variables dependent upon it.



Projects are due tomorrow.  Check yesterday's blog for a list of all items that should be included.  NO LATE PROJECTS will be accepted.

Sections Covered in Textbook:

5-1: Relating Graphs to Events

Resources & Tutorials:

1) Relating Graphs to Events
2) Relating Graphs to Events - another video

3) Relating Graphs to Events class notes

Dice Project - Finishing Up

Today We Discussed:

We used today's class to finish our discussion about probability, and to work on finishing projects.  All students are encouraged to type their conclusions using Google Docs.  Reminder, the finished project should include the following items:

• Rubric
• Printout of Data from 3 Trials of Rolling Dice
• Summary of Trial Data
• Frequency Tables (4)
• Theoretical Outcomes
• Histogram Containing 3 Trials
• Histogram of Trial Averages vs Theoretical Outcomes
• ThinkSheet
• Written Conclusion (Typed preferred)

Ms. Dause worked with students during study hall on how to effectively write a project conclusion.  

Sections Covered in Textbook:

None

Resources & Tutorials:

1) Project Packet (if needed)
2) Discussion Questions (if needed)

Dice Project - Chart Work Day

Today We Discussed:

We continued our progress with our dice project, and learned how to create new charts from our collected data.  For this project, we only focused on histograms/bar charts/column charts.  The completed project will have two histograms that are outlined in the project packet.

We also reviewed some practical uses of spreadsheets to gain better understanding of how and why they are used.

Finally, we discussed some of the things we learned by completing this project.  Students were given a list of discussion questions that they should use to build their conclusion document to be included with the final project. We will spend some time in class tomorrow going over the discussion questions and comparing data with each other.  

Sections Covered in Textbook:

None

Resources & Tutorials:

1)  Class Notes:  How to Create Charts in GSheets.
2)  How to create a column chart in GSheets (Video).
3)  Dice Project Discussion Questions

Chapter 4 Review and Frequency Tables

Topics for Today:

Today we reviewed briefly for our test tomorrow on Chapter 4.

We also developed our sample space and learned how to use frequency tables to organize our theoretical and experimental data from our project.

We have a test tomorrow, and notebooks are due.

By Wednesday, you need to have all of your data tallied and totaled on your frequency tables.

Attached is a copy of the review sheet, and the answers. Good luck!

Sections Covered in Textbook:

No new sections covered.  

Resources & Tutorials:

1) Chapter 4 Review Sheet

2) Chapter 4 Review Sheet answers

Probability of Compound Events

Today We Discussed:

Today we discussed probability of compound events.  We reviewed what the word "compound" means (and in this case, it simply means more than one), and discussed the different types of compound events.  There are two types:  independent events and dependent events.  Independent events occur when the outcome of one event has no effect on the other (ex:  flipping a coin twice).  Dependent events occur when the outcome of the first event does have an effect on the event that comes after (ex:  taking a card from a deck of cards and keeping it, then taking another card).

The numeric probability of dependent events can be found by multiplying the theoretical probability of each event together.

For two independent events, P(A and B) = P(A) * P(B)
For two dependent events, P(A then B) = P(A) * P(B after A)

Vocabulary: independent events, dependent events

Sections Covered in Textbook:

4-6: Probability of Compound Events

Resources & Tutorials:

1) What are compound events?
2) How to determine if your events are independent or dependent.
3) How to find probability of independent events.
4) How to find probability of dependent events.

5) Probability of Compound Events notes

Applying Ratios to Probability

Today We Discussed:

Ratios can be used to express everyday activities, and we will be using them in the context of probability.  Probability simply is the chance that an event can occur.  We defined all of the terms associated with both theoretical and experimental probability, and talked about how to find a sample space and how that relates to probability.  Exploring experimental probability is a good way to demonstrate that what we expect to happen, does not always occur, and probability is just based upon how likely something is to occur, not a guarantee it will occur.

We will be investigating experimental probability more thoroughly in the next week.


Graphic Credit:  Online Math Learning

Vocabulary: probability, outcome, event, sample space, theoretical probability, experimental probability 

Sections Covered in Textbook:

4-5: Applying Ratios to Probability (pages 211-217)

Resources & Tutorials:

1) What is probability?
2) What is an outcome?
3) What is a sample space? 
4) How do you find the probability of a simple event?
5) What is experimental probability?
6) Math is Fun - Probability (not a video)
7) Applying Ratios to Probability Class notes

Percent of Change

Today We Discussed:

We continued the topic of percents in the context of percent of change.  We can have a positive percent of change (representing an increase) or a negative rate of change (representing a decrease).  To determine the percent of change, we have to compare how much something changed to its original quantity.  Percent of change is relative to the original value.

Vocabulary: percent of change, percent of increase, percent of decrease

Sections Covered in Textbook:

4-4: Percent of Change (pages 204-209)

Resources & Tutorials:

1) What is the percent of change?
2) How do you find percent of change?
3) How do you determine percent of increase or decrease?

4) Percent of Change Class notes

Proportions and Percent Equations

Today We Discussed:

We expanded our discussion of proportions to include the percent proportion.  We deconstructed the word per-cent to mean "out of 100".  We can solve percent problems using a proportion or using a percent equation with the percent expressed as a decimal.

This unit will continue to explore proportions and percents, and we'll take some time to review the conversions of fractions to decimals to percents and vice-versa.  Students will be encouraged to memorize the decimal equivalents of common fractions as a time-saver.  Normally I am not a big fan of memorization, unless it serves a useful purpose - memorizing common concepts (like divisibility rules, the quadratic formula, and common numbers) can be a big time-saver leaving more time for higher order problem solving.

As we continue to explore the relationships among decimals, fractions, and percents, I plan to expand our discussion to other proportions, like circles.  We will investigate some probability in this unit as well.

Vocabulary: percent proportion, percent equation

Sections Covered in Textbook:

4-3: Proportions and Percent Equations (pages 197-202)

Resources & Tutorials:

1) What is a percent proportion?
2) How do you use a proportion to find a whole?
3) How do you use a proportion to find what percent a part is of a whole?
4) How do you use a proportion to find part of a whole?
5) What is a percent equation?
6) More Percent Equation Links

7) Proportion and Percent Equations Notes

Ratio and Proportion

Today We Discussed:

We began our unit on solving and applying proportions today, and introduced/reviewed some important vocabulary, beginning with ratios.  A ratio is just a comparison of numbers by division.  Students have seen ratios ever since they began working with fractions.  When we talk about rates, we create a ratio of two numbers that have different units.  We have already seen rates this year, when dealing with uniform motion -  rate of speed (comparing a distance with how much time elapses). 

We also used conversion factors to convert rates.  A conversion factor is a rate that is equal to 1 (multiplicative identity states we can multiply by 1 and not change the identity of our number).   For example, a unit conversion would be 60 seconds per minute since 1 minute=60 seconds.

Finally, we used the means-extremes (cross products) property to solve proportions. 

Vocabulary: ratio, rate, unit rate, conversion factor, unit analysis, dimensional analysis, proportion, cross products

Sections Covered in Textbook:

4-1: Ratio and Proportion (pages 182-187)

Resources & Tutorials:

1) What is a ratio?
2) What are rates and unit rates?
3) What is dimensional or unit analysis?
4) What is a proportion?
5) How to solve a proportion by using cross products?

6) Ratio and Proportion Notes

Chapter 3 Review

Today We Discussed:

Today we reviewed the topics from Chapter 3 in preparation for our test on Thursday. 

Our chapter covered the following items:

• Inequalities
• Multi-step
• Compound (AND and OR)
• Graphing
• Absolute Value
• Equations
• Inequalities

The big "take-aways" from this chapter are:

• Equations have finite solutions; inequalities have infinite solutions.
• When multiplying or dividing an inequality by a negative, you must flip the inequality sign to keep the truth of the inequality.
• Absolute Value equations and inequalities have two cases: positive and negative
• Absolute Value Inequalities come in two forms
• Greater than inequalities generate OR compound inequalities
• Less than inequalities generate AND compound inequalities

Sections Covered in Textbook:

All of Chapter 3 (pages 132-172) 

Resources & Tutorials:

Chapter 3 Review

Chapter 3 Review Answers

Absolute Value Inequalities

Today We Discussed:

We continued our discussion about absolute value but moved on to inequalities.  Just like absolute value equations, we must consider TWO cases for absolute value inequalities - the positive case and the negative case.  Furthermore, we have to analyze which direction our solutions go based upon whether we are dealing with a greater than absolute value inequality or a less than absolute value inequality.

• Greater than absolute value inequalities function like OR compound inequalities.
• Less than absolute value inequalities function like AND compound inequalities.  

Graphic Credit: http://andymath.com/solving-absolute-value-inequalities/

Sections Covered in Textbook:

3-6: Absolute Value Equations and Inequalities (pages 167-171)

Resources & Tutorials:

1) How do you figure out if you have an AND or OR compound inequality?
2) How to solve an AND absolute value equation.
3) Introduction to Absolute Value Inequalities.
   (Use navigation on the left for more types of examples.)

4) Absolute Value Inequalities Notes

Absolute Value Equations

Today We Discussed:

We moved into the next section in our book and discussed absolute value equations.  We will tackle absolute value inequalities on Thursday and Monday.

First, we reviewed absolute value and what it means - a number's positive distance from zero.  Absolute value equations add a small level of complexity because when we take the absolute value of a quantity, it will always be positive.   We can have an expression inside the absolute value bars be either positive OR negative, so we can end up with two solutions for the variable in these cases.

We must also analyze whether or not our absolute value equation makes sense.  In most cases, we will get two solutions, but there will be times when no solutions will be possible.  We need to make sure our equation is logical. 

Take for example the equation |x -2| = -3.   

There will never be a case when we take the absolute value of an expression that will result in a solution that is less than 0.  By its very definition, absolute value is always positive.  

For each of these absolute value equations, we will need to consider TWO cases for each solution set:  the positive case and the negative case. We will need to solve TWO equations to get the complete solution for the variable.

Sections Covered in Textbook:

3-6: Absolute Value Equations and Inequalities (pages 167-171)
(We will only cover equations today!)

Resources & Tutorials:

1) Four steps to solve absolute value equations. 
2) Introduction to absolute value equations.
3) Chili Math - Solving Absolute Value Equations (Not a video)

4) Absolute Value Equations Notes

Compound Inequalities

Topics for Today:

Our discussion about inequalities has moved on to compound inequalities.  We discussed the word "compound" and related it to compound words and compound sentences.  There are two types of compound inequalities:

• inequalities using OR 
• inequalities using AND. 

For the OR types, only part of the inequality needs to be true for the entire compound statement to be true.  For the AND types, we must have both parts true at the same time.  OR inequalities can be related to the UNION of two sets, and AND types represent the INTERSECTION (where both criteria are true at the same time).   Venn Diagrams (circle diagrams) are often used as pictorial representations of our sets.  

Graphic Credit:  

https://rstudio-pubs-static.s3.amazonaws.com/282515_ddb4251ce953466fb8055bb4fd4837b9.html

Vocabulary: compound inequality

Sections Covered in Textbook:

3-5: Compound Inequalities (pages 161-165)

Resources & Tutorials:

1) What is a compound inequality?
2) How do you solve an OR compound inequality and graph it?
3) How do you solve an AND compound inequality and graph it?
4) How do you solve an AND compound inequality rewriting it as two?
5) What is a Venn Diagram?
6) Schoolhouse Rock - Conjunctions

7) Compound Inequalities Notes

Solving Multi-Step Inequalities

Topics for Today:

We continue to build on our problem-solving skills with solving inequalities.  Today we moved on to more complicated inequalities that involve several steps.  Again, we approach these problems just like solving equations, with the first step being to identify the variable.  Once we identify the variable, we need to plan for how we "undo" operations performed on the variable with the goal of getting the variable by itself.  To accomplish this goal, we perform the order of operations (PEMDAS) in reverse.  *Students must always keep in mind that when multiplying or dividing an inequality by a negative number, they must reverse (flip) the inequality sign to keep the truth of the inequality.*

Sections Covered in Textbook:

Solving Multi-Step Inequalities (pages 153-159)

Resources & Tutorials:

1) How do you solve a multi-step inequality?
2) How do you solve an inequality with variables on both sides?
3) How to solve multi-step inequalities.

4) Solving Multi-Step Inequalities Notes

Solving Inequalities Using Multiplication and Division

Topics for Today:

Our discussion about solving inequalities moved to solving by using the multiplication and division properties of inequality.  We solve inequalities using the same steps and procedures as solving equations, but there is one notable exception.  For cases when we either multiply or divide both sides of our inequality by a negative number, we must switch the inequality sign to preserve the truth of the inequality.  To illustrate why this works, we did a little exploration with simple inequalities in class to help understand why the "truth" of an inequality changes.

Vocabulary: multiplication property of inequality, division property of inequality

We considered the following examples in class:

Sections Covered in Textbook:

3-3: Solving Inequalities Using Multiplication & Division
       (pages 146-151)

Resources & Tutorials:

1) What is the division property of inequality?
2) What is the multiplication property of inequality?
3)  Solving inequalities using multiplication and division
4) Virtual Nerd Page with more tutorials.

5)  Solving Inequalities Using Multiplication and Division Notes

Assignments:

1) Solving Inequalities Part II Worksheet

2) Chapter 2 Test Corrections due Wednesday (7th) or Thursday (8th)

Solving Inequalities Using Addition and Subtraction

Topics for Today:

Now that we understand what inequalities and solutions to inequalities are, we can now move into solving them.  When solving simple inequalities using addition and subtraction, we basically follow the same steps we use for solving simple equations.  For these problems, we will use the addition and subtraction properties of inequality to "undo" operations performed on a variable with the goal of getting the variable by itself.  Checking solutions to inequalities may not always locate our mistakes since there are an infinite number of possible solutions we can use to check ourselves.  Students will be encouraged to try out multiple possible solutions when checking.

Vocabulary: equivalent inequalities, addition property of inequality, subtraction property of inequality.

Graphic Credit:
https://www.mathwarehouse.com/number-lines/graph-inequality-on-number-line.php#examples1

Sections Covered in Textbook:

3-2: Solving Inequalities Using Addition and Subtraction (pages 140-144)

Resources & Tutorials:

1) What is the addition property of inequality? 
2) How do you solve an inequality using subtraction?
3) How do you solve an inequality using addition?

    (This includes putting the solution in set notation, which we did not discuss in class.)

4) Solving Inequalities Using Addition and Subtraction Notes

Inequalities and Their Graphs

Topics for Today:

We moved on to Chapter 3 today.  Our discussion has moved from equations where both sides are equal, to inequalities where one side is larger or smaller than the other.  We also discussed the difference between solutions where the endpoint is included vs excluded and explored the graphs of inequalities.  A solution to an inequality is any value that will make the inequality true.  Inequalities differ from equations because inequalities often have infinite solutions that are bound by a particular value whereas equations typically have a finite solution set.

Graphic Credit: https://www.katesmathlessons.com/graphing-inequalities.html

Vocabulary: inequality, solution to an inequality

Sections Covered in Textbook:

3-1: Inequalities and Their Graphs (pages 134-138)

Resources & Tutorials:

1) What is an Inequality?
2) How Do You Graph Inequalities?
    (This video also includes infinite sets which we did not discuss.)

3) Inequalities and Their Graphs Class Notes

Chapter 2 Review

Topics for Today:

In preparation for our Chapter 2 test tomorrow, we spent the majority of our class working through practice problems.  Students should spend time tonight going through old homework and practicing solving and writing equations.  The test will cover solving one-step, two-step, and multi-step equations as well as equations with variables on both sides.  It will also cover problem solving with defining variables in terms of each other, consecutive number problems, break-even problems, and uniform motion (d=rt), and finally, literal equations.  

Study Skills:  Did you run out of problems to practice?  No problem!  Try working out the exercises at the end of each book section - there are example problems worked out in each section, and the homework problems are labeled in green to identify which practice problem is like the problem listed in the exercises.  The odd numbered problems have answers in the back so you can check to make sure you are solving the problems correctly!

You may also go over old Delta Math assignments for many more examples of solving equations. 

Sections Covered in Textbook:

Chapter 2 Test will cover Sections 2-1 through 2-6 (Pages 74-115)

Resources & Tutorials:

1) Chapter 2 Review Sheet

2) Answers to Chapter 2 Review Sheet

Literal Equations

Topics for Today:

Today we continued our exploration of equations, but focused on literal equations and formulas.  Literal equations are just equations that have more than one variable.  Formulas are mathematical or scientific facts, rules, or relationships expressed with mathematical symbols.  Students have been using formulas for much of their mathematics studies, although they may not be aware.  Earlier in this chapter, we used the formula for distance (d=rt) and also perimeter of a rectangle {P=2(l+w)}.

We can use our knowledge of solving equations to move variables around in literal equations or formulas, to solve for a particular variable.  The properties of equality (addition, subtraction, multiplication, division and distributive) still apply here in these examples.

The first step in any solving equation problem it to identify the variable that you are solving for.  Once that is done, we follow the reverse order of operations to isolate the variable, and follow the same steps we used for solving multi-step equations.  As a reminder, here are those steps again:

Vocabulary: formula, literal equation

Sections Covered in Textbook:

2-6: Formulas (pages 111-114)

Resources & Tutorials:

1) What is a literal equation?
2) How do you solve a formula for a variable?
3) Summary of solving literal equations.

4) Literal Equations class notes

Uniform Motion Problems - Equations and Problem Solving Part 2

Topics for Today:

We continued our discussion about problem solving by investigating uniform motion problems.   Most students are familiar with the basic formula for motion: 
distance = rate * time, or in algebraic terms, d = rt.

Uniform motion problems fall into three main categories: same-direction travel, round-trip travel, or opposite-direction travel.  Depending upon what the problem is asking, we'll combine our problem data in different ways to find our answer, but in each case, we will still apply the general formula (d=rt) to set up our problem.

Drawing diagrams to help picture what is going on in the problem is another helpful strategy.  Using a table to solve problems helps organize all the supporting data, and provides a systematic way to solve more complex problems.  Students are encouraged to use a table and to draw a picture of what is going on in the question to help better understand what is being asked.

Sections Covered in Textbook:

2-5: Equations and Problem-Solving Part 2 (pages 103-110)

Resources & Tutorials:

1)  How to Solve Opposite-Directions problem.
2)  How to Solve Same-Directions problem.
3)  How to Solve Round-Trip Travel Problems.
4)  Problems Solving Notes Part 2 - Uniform Motion

Equations and Problem Solving Part 1

Topics for Today:

Now that we know how to solve all types of equations, we will use that knowledge to solve story problems.  There are many different types of story problems, but most of them can be categorized into one of several categories.  Today's lesson will focus on problem solving involving defining variables in terms of each other,  consecutive integers (which also involves defining variables in terms of another), and break-even problems.

Vocabulary: consecutive, break-even

Sections Covered in Textbook:

2-5: Equations and Problem Solving (pages 103 - 110)
We will continue working on this topic tomorrow with more examples.

Resources & Tutorials:

1) Solving Break-Even Problems
2) How to find the Break-Even Point
3) Solving Word Problems with Consecutive Integers
4) Problem Solving Notes Part 1

Solving Equations with Variables on Both Sides

Topics for Today:

Our discussion about solving equations moved on to situations where there are variables on both sides of an equation.  The basic properties of equality still apply when dealing with variable terms.  We used the addition, subtraction, multiplication, and division properties of equality to get variables on one side, and constants on the other side of the equation.  For equations with variables on both sides, it is possible for the equation to have infinitely many solutions (identity) or no solution at all.  Students will need to be on the lookout for these special cases, which show themselves is interesting mathematical ways.

Sections Covered in Textbook:

2-4: Equations with Variables on Both Sides (pages 96-100)

Resources & Tutorials:

1) Solving Equations with Variables on Both Sides
2) Solving Equations with Variables on Both Sides and Grouping Symbols

3) Equations with Variables on Both Sides Class Notes