Solving Absolute Value Inequalities

Today We Discussed:

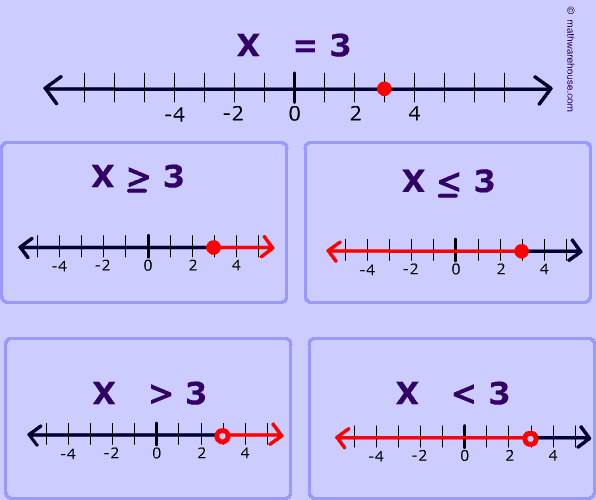
We continued our discussion about absolute value but moved on to inequalities.  Just like absolute value equations, we must consider TWO cases for absolute value inequalities - the positive case and the negative case.  Furthermore, we have to analyze which direction our solutions go based upon whether we are dealing with a greater than absolute value inequality or a less than absolute value inequality.

• Greater than absolute value inequalities function like OR compound inequalities.
• Less than absolute value inequalities function like AND compound inequalities.  

Graphic Credit: http://andymath.com/solving-absolute-value-inequalities/

Sections Covered in Textbook:

3-6: Absolute Value Equations and Inequalities (pages 167-171)

Resources & Tutorials:

1) How do you figure out if you have an AND or OR compound inequality?
2) How to solve an AND absolute value equation.
3) Introduction to Absolute Value Inequalities.
   (Use navigation on the left for more types of examples.)

Solving Absolute Value Equations

Today We Discussed:

We moved into the next section in our book and discussed absolute value equations.  We will tackle absolute value inequalities on Thursday and Monday.

First, we reviewed absolute value and what it means - a number's positive distance from zero.  Absolute value equations add a small level of complexity because when we take the absolute value of a quantity, it will always be positive.   We can have an expression inside the absolute value bars be either positive OR negative, so we can end up with two solutions for the variable in these cases.

We must also analyze whether or not our absolute value equation makes sense.  In most cases, we will get two solutions, but there will be times when no solutions will be possible.  We need to make sure our equation is logical. 

Take for example the equation |x -2| = -3.   

There will never be a case when we take the absolute value of an expression that will result in a solution that is less than 0.  By its very definition, absolute value is always positive.  

For each of these absolute value equations, we will need to consider TWO cases for each solution set:  the positive case and the negative case. We will need to solve TWO equations to get the complete solution for the variable.

Sections Covered in Textbook:

3-6: Absolute Value Equations and Inequalities (pages 167-171)
(We will only cover equations today!)

Resources & Tutorials:

1) Four steps to solve absolute value equations. 
2) Introduction to absolute value equations.
3) Chili Math - Solving Absolute Value Equations (Not a video)

Solving Compound Inequalities

Topics for Today:

Our discussion about inequalities has moved on to compound inequalities.  We discussed the word "compound" and related it to compound words and compound sentences.  There are two types of compound inequalities:

• inequalities using OR 
• inequalities using AND. 

For the OR types, only part of the inequality needs to be true for the entire compound statement to be true.  For the AND types, we must have both parts true at the same time.  OR inequalities can be related to the UNION of two sets, and AND types represent the INTERSECTION (where both criteria are true at the same time).   Venn Diagrams (circle diagrams) are often used as pictorial representations of our sets.  

Graphic Credit:  

https://rstudio-pubs-static.s3.amazonaws.com/282515_ddb4251ce953466fb8055bb4fd4837b9.html

Vocabulary: compound inequality

Sections Covered in Textbook:

3-5: Compound Inequalities (pages 161-165)

Resources & Tutorials:

1) What is a compound inequality?
2) How do you solve an OR compound inequality and graph it?
3) How do you solve an AND compound inequality and graph it?
4) How do you solve an AND compound inequality rewriting it as two?
5) What is a Venn Diagram?
6) Schoolhouse Rock - Conjunctions

Solving Multi-Step Inequalities

Topics for Today:

We continue to build on our problem-solving skills with solving inequalities.  Today we moved on to more complicated inequalities that involve several steps.  Again, we approach these problems just like solving equations, with the first step being to identify the variable.  Once we identify the variable, we need to plan for how we "undo" operations performed on the variable with the goal of getting the variable by itself.  To accomplish this goal, we perform the order of operations (PEMDAS) in reverse.  *Students must always keep in mind that when multiplying or dividing an inequality by a negative number, they must reverse (flip) the inequality sign to keep the truth of the inequality.*

Sections Covered in Textbook:

Solving Multi-Step Inequalities (pages 153-159)

Resources & Tutorials:

1) How do you solve a multi-step inequality?
2) How do you solve an inequality with variables on both sides?
3) How to solve multi-step inequalities.

Solving Inequalities Using Multiplication and Division

Topics for Today:

Our discussion about solving inequalities moved to solving by using the multiplication and division properties of inequality.  We solve inequalities using the same steps and procedures as solving equations, but there is one notable exception.  For cases when we either multiply or divide both sides of our inequality by a negative number, we must switch the inequality sign to preserve the truth of the inequality.  To illustrate why this works, we did a little exploration with simple inequalities in class to help understand why the "truth" of an inequality changes.

Vocabulary: multiplication property of inequality, division property of inequality

We considered the following examples in class:

Sections Covered in Textbook:

3-3: Solving Inequalities Using Multiplication & Division
       (pages 146-151)

Resources & Tutorials:

1) What is the division property of inequality?
2) What is the multiplication property of inequality?
3)  Solving inequalities using multiplication and division
4) Virtual Nerd Page with more tutorials.

Solving Inequalities Using Addition and Subtraction

Topics for Today:

Now that we understand what inequalities and solutions to inequalities are, we can now move into solving them.  When solving simple inequalities using addition and subtraction, we basically follow the same steps we use for solving simple equations.  For these problems, we will use the addition and subtraction properties of inequality to "undo" operations performed on a variable with the goal of getting the variable by itself.  Checking solutions to inequalities may not always locate our mistakes since there are an infinite number of possible solutions we can use to check ourselves.  Students will be encouraged to try out multiple possible solutions when checking.

Vocabulary: equivalent inequalities, addition property of inequality, subtraction property of inequality.

Graphic Credit:
https://www.mathwarehouse.com/number-lines/graph-inequality-on-number-line.php#examples1

Sections Covered in Textbook:

3-2: Solving Inequalities Using Addition and Subtraction (pages 140-144)

Resources & Tutorials:

1) What is the addition property of inequality? 
2) How do you solve an inequality using subtraction?
3) How do you solve an inequality using addition?

    (This includes putting the solution in set notation, which we did not discuss in class.)

Inequalities and Their Graphs

Topics for Today:

We moved on to Chapter 3 today.  Our discussion has moved from equations where both sides are equal, to inequalities where one side is larger or smaller than the other.  We also discussed the difference between solutions where the endpoint is included vs excluded and explored the graphs of inequalities.  A solution to an inequality is any value that will make the inequality true.  Inequalities differ from equations because inequalities often have infinite solutions that are bound by a particular value whereas equations typically have a finite solution set.

Graphic Credit: https://www.katesmathlessons.com/graphing-inequalities.html

Vocabulary: inequality, solution to an inequality

Sections Covered in Textbook:

3-1: Inequalities and Their Graphs (pages 134-138)

Resources & Tutorials:

1) What is an Inequality?
2) How Do You Graph Inequalities?
    (This video also includes infinite sets which we did not discuss.)

Literal Equations

Topics for Today:

Today we continued our exploration of equations, but focused on literal equations and formulas.  Literal equations are just equations that have more than one variable.  Formulas are mathematical or scientific facts, rules, or relationships expressed with mathematical symbols.  Students have been using formulas for much of their mathematics studies, although they may not be aware.  Earlier in this chapter, we used the formula for distance (d=rt) and also perimeter of a rectangle {P=2(l+w)}.

We can use our knowledge of solving equations to move variables around in literal equations or formulas, to solve for a particular variable.  The properties of equality (addition, subtraction, multiplication, division and distributive) still apply here in these examples.

The first step in any solving equation problem it to identify the variable that you are solving for.  Once that is done, we follow the reverse order of operations to isolate the variable, and follow the same steps we used for solving multi-step equations.  As a reminder, here are those steps again:

Vocabulary: formula, literal equation

Sections Covered in Textbook:

2-6: Formulas (pages 111-114)

Resources & Tutorials:

1) What is a literal equation?
2) How do you solve a formula for a variable?
3) Summary of solving literal equations.

Equations and Problem Solving Part 2 - Uniform Motion

Topics for Today:

We continued our discussion about problem solving by investigating uniform motion problems.   Most students are familiar with the basic formula for motion: 
distance = rate * time, or in algebraic terms, d = rt.

Uniform motion problems fall into three main categories: same-direction travel, round-trip travel, or opposite-direction travel.  Depending upon what the problem is asking, we'll combine our problem data in different ways to find our answer, but in each case, we will still apply the general formula (d=rt) to set up our problem.

Drawing diagrams to help picture what is going on in the problem is another helpful strategy.  Using a table to solve problems helps organize all the supporting data, and provides a systematic way to solve more complex problems.  Students are encouraged to use a table and to draw a picture of what is going on in the question to help better understand what is being asked.

Sections Covered in Textbook:

2-5: Equations and Problem-Solving Part 2 (pages 103-110)

Resources & Tutorials:

1)  How to Solve Opposite-Directions problem.
2)  How to Solve Same-Directions problem.
3)  How to Solve Round-Trip Travel Problems.

Equations and Problem Solving Part 1

Topics for Today:

Now that we know how to solve all types of equations, we will use that knowledge to solve story problems.  There are many different types of story problems, but most of them can be categorized into one of several categories.  Today's lesson will focus on problem solving involving defining variables in terms of each other,  consecutive integers (which also involves defining variables in terms of another), and break-even problems.

Vocabulary: consecutive, break-even

Sections Covered in Textbook:

2-5: Equations and Problem Solving (pages 103 - 110)
We will continue working on this topic tomorrow with more examples.

Resources & Tutorials:

1) Solving Break-Even Problems
2) How to find the Break-Even Point
3) Solving Word Problems with Consecutive Integers

Solving Equations with Variables on Both Sides

Topics for Today:

Our discussion about solving equations moved on to situations where there are variables on both sides of an equation.  The basic properties of equality still apply when dealing with variable terms.  We used the addition, subtraction, multiplication, and division properties of equality to get variables on one side, and constants on the other side of the equation.  For equations with variables on both sides, it is possible for the equation to have infinitely many solutions (identity) or no solution at all.  Students will need to be on the lookout for these special cases, which show themselves is interesting mathematical ways.

Sections Covered in Textbook:

2-4: Equations with Variables on Both Sides (pages 96-100)

Resources & Tutorials:

1) Solving Equations with Variables on Both Sides
2) Solving Equations with Variables on Both Sides and Grouping Symbols

Solving Multi-Step Equations

Topics for Today:

We moved on from two-step to multi-step equation solving.  Today's lesson focused on simplifying with grouping symbols, and multiplying through to eliminate fractions and decimals to make the equation easier to solve.

Sections Covered in Textbook:

2-3: Solving Multi-Step Equations (pages 88 - 93)

Resources & Tutorials:

1) Solving Multi-Step Equations using Distributive Property
2) Solving Multi-Step Equations by Clearing Fractions

Solving Two-Step Equations

Topics for Today:

Our discussion about solving equations moved on to solving 2-step equations.  The order of operations still figures in to this process, although since we are undoing operations, we go in reverse.

Sections Covered in Textbook:

2-2: Solving Two-Step Equations (pages 81- 86)

Resources & Tutorials:

1) How do you solve a 2-step equation?
2) Math Antics - How to solve 2-step equations.

Solving One-Step Equations

Topics for Today:

Today we reviewed solving one-step algebraic equations.  We discussed what inverse operations are, and also defined the term solution.  Students are reminded that all they are accountable for all the mathematics that came before this class, as well as any new learned material.  Mathematics is a cumulative subject, and the skills built in the past will continue to be used to solve new problems.  That means that we will continue to integrate fraction and decimal operations into our problem solving.  Equation operations will include all types of real numbers.

Sections Covered in Textbook:

2-1: Solving One-Step Equations (pages 74-79)

Resources & Tutorials:

1) Math Antics - How to Solve a 1-step Equation.
2) How to solve an equation using addition.
3) How to solve an equation using subtraction.
4) How to solve an equation using multiplication.
5) How to solve an equation using division.
6) How to solve a decimal equation using subtraction.
7) How to solve an equation when multiplying fractions. 

Chapter 1 Review

Topics for Today:

Today we played a game of Money Grab to finish up our review of Chapter 1.  Students should make sure their binders are fully organized prior to joining class on Thursday.  Binders will be collected at the beginning of class.  For a list of topics that need to be in the notebook, open the Chapter 1 Table of Contents Google Docs that I have shared with you from the Chapter 1 folder.  

Topics covered in Chapter 1:  Using variables, order of operations, classifications of real numbers, real number operations (adding, subtracting, multiplying, and dividing), as well as the properties of equality.  

Sections Covered in Textbook:

Chapter 1:  Tools of Algebra (pages 4-58) - Omit section 1-9

Resources & Tutorials:

The Distributive Property

Topics for Today:

Today we discussed the distributive property  of multiplication over addition and subtraction.  We also used the distributive property to multiply large numbers using mental math.  We also discussed how to combine algebraic like terms, and expanded our algebra vocabulary.  We revisited translating phrases into algebraic expressions with the addition of the distributive property.

Vocabulary: constant, like terms, distributive property, coefficient, term

Sections Covered in Textbook:

1-7: The Distributive Property (pages 47 - 52)

Resources & Tutorials:

1) What is the distributive property?

Multiplying and Dividing Real Numbers

Topics for Today:

Earlier this week we took our first quiz of the year.  How did it go?  Did you properly prepare?  If not, what improvements can you make for the future?  Students will be allowed and expected to complete quiz corrections for partial credit.  Quizzes are meant as assessment and learning tools.  Students should always be asking, "How can I do better?".

We moved on to multiplying and dividing real numbers today.

Some basic sign rules for multiplication and division:

+ · + = +      + / + = +
+ · - = -      + / - = -
- · + = -      - / + = -
- · - = +      - / - = +

Vocabulary: Identity property of Multiplication, Multiplication Property of Zero, Multiplication Property of -1, Inverse Property of Multiplication, multiplicative inverse, reciprocal

Sections Covered in Textbook:

1-6: Multiplying and Dividing Real Numbers (pages 37 - 44)

Resources & Tutorials:

1) How do you multiply and divide numbers with different signs?
2) What are Multiplicative Inverses?
3) What is a reciprocal?

Subtracting Real Numbers

Topics for Today:

We continued our discussion of adding real numbers (both positive and negative) using a number line and integer chips.  Our rules for addition are:

• If the signs are the same, add and keep the sign.
• If the signs are different, subtract and keep the sign of the number with the larger absolute value.  

Stated another way...

Subtraction is actually the addition of opposites!  

For subtraction problems, first change to addition by adding the opposite, then follow your addition rules!  

                  6 – 10 =

              6 + (-10) =  

Subtract 6 from 10 and keep the negative sign because |-10| > |6|

Therefore, the answer is – 4. 

Sections Covered in Textbook:

1-4: Adding Real Numbers (pages 24-31)
1-5: Subtracting Real Numbers (pages 32-36)

Resources & Tutorials:

1) How do you add two negative numbers?
2) Rules for Adding Integers
3)  How to rewrite a subtraction problem as addition

Adding Real Numbers

Topics for Today:

We used models (number line and integer chips) and rules to add real numbers today.  We talked about the identity property of addition and also talked about inverses.  We also discussed how to use a number line to represent addition problems of both positive and negative integers.  


Vocabulary: identity property of equality, additive inverse, inverse property of addition

Sections Covered in Textbook:

1-4: Adding Real Numbers (pages 24-31)

Resources & Tutorials:

1) How do you add two negative numbers?
2) Rules for Adding Integers
3) What is the opposite of a number?
4) What are identities?

Exploring Real Numbers

Topics for Today:

We are still working on the language of Algebra and using precise words.  Today we discussed the different classifications for numbers and what sets various types of numbers belong to (Natural, Whole, Integers, Rational, Irrational, and Real).  We also reviewed the meaning of absolute value.

Vocabulary: natural numbers, whole numbers, integers, rational number, irrational number, real numbers, inequality, opposites, absolute value

Sections Covered in Textbook:

1-3: Exploring Real Numbers (Pages 17-23)

Resources & Tutorials:

1) What is a real number?
2) What is a rational number?
3) What are natural and whole numbers?

Exponents and Order of Operations

Topics for Today:

As with any language, algebra has some basic rules that must be followed to arrive at a correct answer.  Sometimes there are multiple ways to express an answer or number so we need a set of rules to follow so we always arrive at the same answer for any one particular problem.  The order of operations is a set of rules that tells problem solvers which operations to perform and in what order.

Vocabulary: simplify, exponent, base, power, order of operations (PEMDAS), evaluate 

Sections Covered in Textbook:

1-2: Exponents and Order of Operations (pages 9 - 16)

Resources & Tutorials:

1) What is the order of operations?
2) How do you use the order of operations

Writing Variable Expressions

Topics for Today:

Our Algebra lesson focused on some of the language of Algebra and how words can be translated into algebraic expressions.

Image Credit: https://www.pinterest.com/pin/651473902320727044/

Vocabulary:  variable, algebraic expression, equation, open sentence

Sections Covered in Textbook:

1-1: Using Variables (Pages 4-8)

Resources & Tutorials:

1) What are numerical and algebraic expressions?
2) Translating mathematical expressions

Solving Radical Equations

Topics for Today:

We added to our equation solving tools today by working with equations containing radicals.  To solve these equations, we must isolate the variable on one side of the equation.  Once we do that, we can "undo" taking a square root by squaring both sides.  We must be careful when squaring equations so that our process does not result in extraneous (extra) solutions.  It's always best to check our solutions to make sure they satisfy the original equation.  As with many other equation types, we may have a situation where our equation has no solutions.  In Algebra I, we do not work with imaginary numbers (in our class they are the square roots of negative numbers), so if we encounter any of these, our equation has no real solution.

Vocabulary: radical equation, extraneous solution

Sections Covered in Textbook:

11-5: Solving Radical Equations (pages 607-612)

Resources & Tutorials:

1) How do you solve radical equations?
2) How to solve a radical equation with a binomial radicand.

Operations with Radicals and Other Roots

Topics for Today:

We finished our discussion of operations with radical expressions today with a method to simplify fractions with radical operations in the denominator.  We discussed the topic of conjugates to rationalize denominators that fall into this category.

We also discussed different roots other than square roots, and how to find them.

Vocabulary:  conjugate, cube root

Sections Covered in Textbook:

11-4:  Operations with Radical Expressions (pages 600-605)
**Other Root Functions are not in our book.

Resources & Tutorials:

1) Divide by Conjugate Method
2) Math is Fun: Cubes and Cube Roots (not a video).
3) How do you find the cube root of a perfect cube? 
4) Fourth Roots

Operations with Radical Expressions Part 1

Topics for Today:

Radicals have some similar properties as variables when we manage them in equations and expressions.  Just like variables, we can only combine radicals that are like each other.  When we combine or take away (add or subtract) radicals, we may only do so if our radicals are like each other.

We can only combine like radicals, and sometimes we need to simplify first, and then we may have like radicals that we can combine.  

The distributive property also works with radicals, including double distributing (otherwise known as FOIL).  

Vocabulary: like radicals, unlike radicals

Sections Covered in Textbook:

11-4: Operations with Radical Expressions (pages 600-606)

Resources & Tutorials:

1) How to add radicals together with like radicands?
2) How do you subtract radicals with like radicands? 
3) How do you subtract radicals with different radicands? 
4) How to use the distributive property with radicals?
5) How to "FOIL" with radicals
6) Divide by Conjugate Method (will do tomorrow)